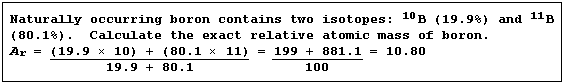REFERENCE SECTION: INTRODUCTION - CHEMICAL CALCULATIONS (1)
Each generation of students is invariably treated to a version of the
following 'words of wisdom': "In my day, everybody knew their tables,
could spell correctly, and knew the capital of each country." Although
there is no evidence that Christopher Robin always went to Pooh-Bear's
tea-parties in those halcyon days of yore, it is worth accepting that
most fables do have grains of truth.
Certainly, it is true that calculations have proven to be the Achilles'
heel for a significant proportion of students. No investigation appears
to have been conducted which might cast light on the reasons for their
difficulties, but one can hazard the suggestion that such students may
have deferred the execution of calculations until late in their course.
Be that as it may, confidence in this topic can be acquired as follows:
beg, borrow, or buy a copy of J. A. Hunt and A. Sykes' well-structured
book (Chemistry Calculations, Longman, London, 1985); and then, from
the outset of the course, work steadily through it. The scope of the
text below is much more modest: primarily, the provision of a reference
set of examples of the commonest types of calculations.

Isotopes
A Periodic Table shows each element's atomic number; e.g., carbon has
the atomic number 6 (because each carbon atom contains 6 protons in its
nucleus). However, such a Table does not normally show that there is 
usually more than one type of atom for each element; e.g., there are 
three types of carbon atom: carbon-12 (¹²C), which contains 6 neutrons; 
carbon-13 (¹³C), with 7 neutrons; and carbon-14, with 8 neutrons.

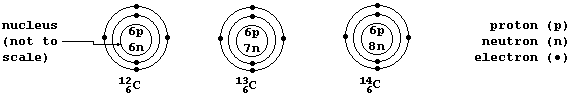
Isotopes are atoms of the same element that contain different numbers
of neutrons. So, C-12, C-13, and C-14 are the three isotopes of carbon.

The Mole
The mole is defined as 'the amount of substance that contains as many
elementary entities as there are atoms in exactly 12 g of the carbon-12
isotope'; these entities may be atoms, ions (charged atoms), molecules,
or other particles. Because the number of atoms in 12.00 g of carbon-12
has been determined experimentally as 6.022 × 10²³ (often referred to
as Avogadro's constant), one mole is equal to 6.022 × 10²³ particles.
The careful study of the Table below should prove rewarding.

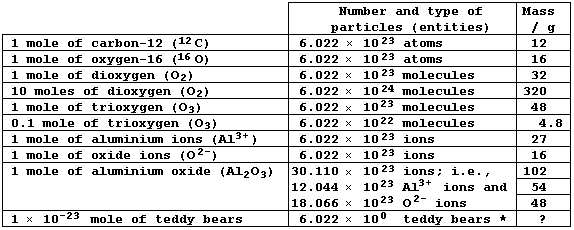
*  The seventh bear is, presumably, little more than a bit of fluff.

Relative Atomic Mass (Ar)
In Nature, elements usually exist as a mixture of isotopes. The (exact)
relative atomic mass of an element is a weighted mean of the relative
isotopic masses of the different isotopes; weighted, that is, in the
proportions in which they occur naturally.

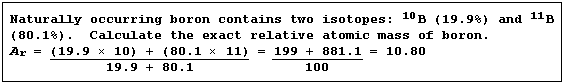
In some versions of the Periodic Table, the exact relative atomic mass 
is given for each element; e.g., 35.4527 for chlorine. In others, each
value is approximated to the nearest whole number: though not that for
chlorine, which is shown as 35.5. There is absolutely no mathematical 
or chemical justification for singling out chlorine; indeed, to be 
brutally frank, the decision to do so was ill-judged. A trawl through 
the exact masses of the elements reveals that roughly a quarter of them
warrant the appendage '.5', including nickel (58.69), copper (63.55), 
zinc (65.39), gallium (69.72), and germanium (72.61).

Relative Molecular Mass (Mr)
The relative molecular mass (Mr) of a molecular element, of a covalent
compound, or of an ionic compound, is calculated by adding together the 
(approximate) relative atomic masses in the molecular formula.

*  Allotropes are two or more forms of the same element that differ in
both chemical and physical properties; e.g., three of the allotropes of
carbon are graphite, diamond, and buckminsterfullerene.

Dr. R. Peters                   Next                     Contents' List